The Mean Value Theorem: Learn It 3

Corollaries of the Mean Value Theorem

Let’s now look at three corollaries of the Mean Value Theorem. These results have important consequences, which we use in upcoming sections.

At this point, we know the derivative of any constant function is zero. The Mean Value Theorem allows us to conclude that the converse is also true. In particular, if [latex]f^{\prime}(x)=0[/latex] for all [latex]x[/latex] in some interval [latex]I[/latex], then [latex]f(x)[/latex] is constant over that interval. This result may seem intuitively obvious, but it has important implications that are not obvious, and we discuss them shortly.

Corollary 1: functions with a derivative of zero

Let [latex]f[/latex] be differentiable over an interval [latex]I[/latex]. If [latex]f^{\prime}(x)=0[/latex] for all [latex]x \in I[/latex], then [latex]f(x)[/latex] is constant for all [latex]x \in I[/latex].

Proof

Since [latex]f[/latex] is differentiable over [latex]I[/latex], [latex]f[/latex] must be continuous over [latex]I[/latex]. Suppose [latex]f(x)[/latex] is not constant for all [latex]x[/latex] in [latex]I[/latex]. Then there exist [latex]a,b \in I[/latex], where [latex]a \ne b[/latex] and [latex]f(a) \ne f(b)[/latex]. Choose the notation so that [latex]a

[latex]\dfrac{f(b)-f(a)}{b-a} \ne 0[/latex]

 

Since [latex]f[/latex] is a differentiable function, by the Mean Value Theorem, there exists [latex]c \in (a,b)[/latex] such that

[latex]f^{\prime}(c)=\dfrac{f(b)-f(a)}{b-a}[/latex]

 

Therefore, there exists [latex]c \in I[/latex] such that [latex]f^{\prime}(c) \ne 0[/latex], which contradicts the assumption that [latex]f^{\prime}(x)=0[/latex] for all [latex]x \in I[/latex].

[latex]_\blacksquare[/latex]

From the example above, it follows that if two functions have the same derivative, they differ by, at most, a constant.

Corollary 2: constant difference theorem

If [latex]f[/latex] and [latex]g[/latex] are differentiable over an interval [latex]I[/latex] and [latex]f^{\prime}(x)=g^{\prime}(x)[/latex] for all [latex]x \in I[/latex], then [latex]f(x)=g(x)+C[/latex] for some constant [latex]C[/latex].

Proof

Let [latex]h(x)=f(x)-g(x)[/latex]. Then, [latex]h^{\prime}(x)=f^{\prime}(x)-g^{\prime}(x)=0[/latex] for all [latex]x \in I[/latex]. By Corollary 1, there is a constant [latex]C[/latex] such that [latex]h(x)=C[/latex] for all [latex]x \in I[/latex]. Therefore, [latex]f(x)=g(x)+C[/latex] for all [latex]x \in I[/latex].

[latex]_\blacksquare[/latex]

The third corollary of the Mean Value Theorem discusses when a function is increasing and when it is decreasing.

Recall that a function [latex]f[/latex] is increasing over [latex]I[/latex] if [latex]f(x_1) < f(x_2)[/latex] whenever [latex]x_1 < _2[/latex], whereas [latex]f[/latex] is decreasing over [latex]I[/latex] if [latex]f(x_1) > f(x_2)[/latex] whenever [latex]x_1 < x_2[/latex].

Using the Mean Value Theorem, we can show that if the derivative of a function is positive, then the function is increasing; if the derivative is negative, then the function is decreasing (Figure 9).

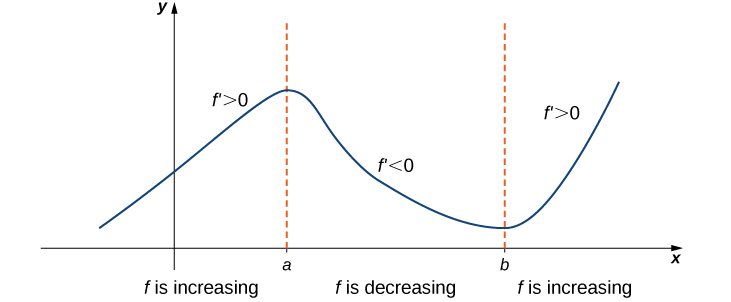
A vaguely sinusoidal function f(x) is graphed. It increases from somewhere in the second quadrant to (a, f(a)). In this section it is noted that f’ > 0. Then in decreases from (a, f(a)) to (b, f(b)). In this section it is noted that f’ < 0. Finally, it increases to the right of (b, f(b)) and it is noted in this section that f’ > 0.
Figure 9. If a function has a positive derivative over some interval [latex]I[/latex], then the function increases over that interval [latex]I[/latex]; if the derivative is negative over some interval [latex]I[/latex], then the function decreases over that interval [latex]I[/latex].

This fact is important because it means that for a given function [latex]f[/latex], if there exists a function [latex]F[/latex] such that [latex]F^{\prime}(x)=f(x)[/latex]; then, the only other functions that have a derivative equal to [latex]f[/latex] are [latex]F(x)+C[/latex] for some constant [latex]C[/latex]. We discuss this result in more detail later in the chapter.

Corollary 3: increasing and decreasing functions

Let [latex]f[/latex] be continuous over the closed interval [latex][a,b][/latex] and differentiable over the open interval [latex](a,b)[/latex].

  1. If [latex]f^{\prime}(x)>0[/latex] for all [latex]x \in (a,b)[/latex], then [latex]f[/latex] is an increasing function over [latex][a,b][/latex].
  2. If [latex]f^{\prime}(x)<0[/latex] for all [latex]x \in (a,b)[/latex], then [latex]f[/latex] is a decreasing function over [latex][a,b][/latex].

Proof

We will prove 1.; the proof of 2. is similar. Suppose [latex]f[/latex] is not an increasing function on [latex]I[/latex]. Then there exist [latex]a[/latex] and [latex]b[/latex] in [latex]I[/latex] such that [latex]a

[latex]f^{\prime}(c)=\dfrac{f(b)-f(a)}{b-a}[/latex]

 

Since [latex]f(a) \ge f(b)[/latex], we know that [latex]f(b)-f(a) \le 0[/latex]. Also, [latex]a0[/latex]. We conclude that

[latex]f^{\prime}(c)=\dfrac{f(b)-f(a)}{b-a} \le 0[/latex]

 

However, [latex]f^{\prime}(x)>0[/latex] for all [latex]x \in I[/latex]. This is a contradiction, and therefore [latex]f[/latex] must be an increasing function over [latex]I[/latex].

[latex]_\blacksquare[/latex]