Substitution

1.  
2. [latex]u=h(x)[/latex]
3. [latex]du=8xdx;f(u)=\frac{1}{8\sqrt{u}}[/latex]
4. [latex]\frac{1}{5}{(x+1)}^{5}+C[/latex]
5. [latex]-\frac{1}{12{(3-2x)}^{6}}+C[/latex]
6. [latex]\sqrt{{x}^{2}+1}+C[/latex]
7. [latex]\frac{1}{8}{({x}^{2}-2x)}^{4}+C[/latex]
8. [latex]\sin \theta -\frac{{ \sin }^{3}\theta }{3}+C[/latex]
9. [latex]\frac{{(1-x)}^{101}}{101}-\frac{{(1-x)}^{100}}{100}+C[/latex]
10. [latex]-\frac{1}{22(7-11{x}^{2})}+C[/latex]
11. [latex]-\frac{{ \cos }^{4}\theta }{4}+C[/latex]
12. [latex]-\frac{{ \cos }^{3}(\pi t)}{3\pi }+C[/latex]
13. [latex]-\frac{1}{4}\phantom{\rule{0.05em}{0ex}}{ \cos }^{2}({t}^{2})+C[/latex]
14. [latex]-\frac{1}{3({x}^{3}-3)}+C[/latex]
15. [latex]-\frac{2({y}^{3}-2)}{3\sqrt{1-{y}^{3}}}[/latex]
16. [latex]\frac{1}{33}{(1-{ \cos }^{3}\theta )}^{11}+C[/latex]
17. [latex]\frac{1}{12}{({ \sin }^{3}\theta -3{ \sin }^{2}\theta )}^{4}+C[/latex]
18. [latex]{L}_{50}=-8.5779.[/latex] The exact area is [latex]\frac{-81}{8}[/latex]
19. [latex]{L}_{50}=-0.006399[/latex] … The exact area is [latex]0[/latex].
20. [latex]u=1+{x}^{2},du=2xdx,\frac{1}{2}{\displaystyle\int }_{1}^{2}{u}^{-1\text{/}2}du=\sqrt{2}-1[/latex]
21. [latex]u=1+{t}^{3},du=3{t}^{2}du,\frac{1}{3}{\displaystyle\int }_{1}^{2}{u}^{-1\text{/}2}du=\frac{2}{3}(\sqrt{2}-1)[/latex]
22. [latex]u= \cos \theta ,du=\text{−} \sin \theta d\theta ,{\displaystyle\int }_{1\text{/}\sqrt{2}}^{1}{u}^{-4}du=\frac{1}{3}(2\sqrt{2}-1)[/latex]
23. 
    The antiderivative is [latex]y= \sin (\text{ln}(2x)).[/latex] Since the antiderivative is not continuous at [latex]x=0,[/latex] one cannot find a value of C that would make [latex]y= \sin (\text{ln}(2x))-C[/latex] work as a definite integral.
24. 
    The antiderivative is [latex]y=\frac{1}{2}\phantom{\rule{0.05em}{0ex}}{ \sec }^{2}x.[/latex] You should take [latex]C=-2[/latex] so that [latex]F(-\frac{\pi }{3})=0.[/latex]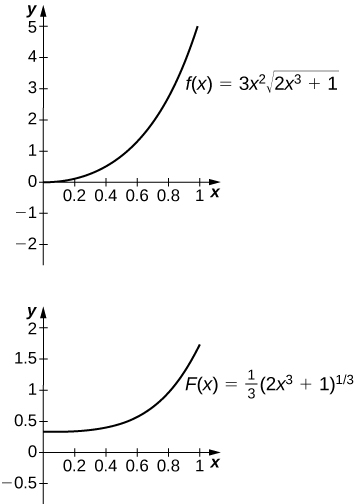
25. 
    The antiderivative is [latex]y=\frac{1}{3}{(2{x}^{3}+1)}^{3\text{/}2}.[/latex] One should take [latex]C=-\frac{1}{3}.[/latex]
26. No, because the integrand is discontinuous at [latex]x=1.[/latex]
27. [latex]u= \sin ({t}^{2});[/latex] the integral becomes [latex]\frac{1}{2}{\displaystyle\int }_{0}^{0}udu.[/latex]
28. [latex]u=(1+{(t-\frac{1}{2})}^{2});[/latex] the integral becomes [latex]\text{−}{\displaystyle\int }_{5\text{/}4}^{5\text{/}4}\frac{1}{u}du.[/latex]
29. [latex]u=1-t;[/latex] the integral becomes

    [latex]\begin{array}{l}{\displaystyle\int }_{1}^{-1}u \cos (\pi (1-u))du\hfill \\ ={\displaystyle\int }_{1}^{-1}u\left[ \cos \pi \cos u- \sin \pi \sin u\right]du\hfill \\ =\text{−}{\displaystyle\int }_{1}^{-1}u \cos udu\hfill \\ ={\displaystyle\int }_{-1}^{1}u \cos udu=0\hfill \end{array}[/latex]
    since the integrand is odd.

30. Setting [latex]u=cx[/latex] and [latex]du=cdx[/latex] gets you [latex]\frac{1}{\frac{b}{c}-\frac{a}{c}}{\displaystyle\int }_{a\text{/}c}^{b\text{/}c}f(cx)dx=\frac{c}{b-a}{\displaystyle\int }_{u=a}^{u=b}f(u)\frac{du}{c}=\frac{1}{b-a}{\displaystyle\int }_{a}^{b}f(u)du.[/latex]
31. [latex]{\displaystyle\int }_{0}^{x}g(t)dt=\frac{1}{2}{\displaystyle\int }_{u=1-{x}^{2}}^{1}\frac{du}{{u}^{a}}=\frac{1}{2(1-a)}{u}^{1-a}{|}_{u=1-{x}^{2}}^{1}=\frac{1}{2(1-a)}(1-{(1-{x}^{2})}^{1-a}).[/latex] As [latex]x\to 1[/latex] the limit is [latex]\frac{1}{2(1-a)}[/latex] if [latex]a<1,[/latex] and the limit diverges to +∞ if [latex]a>1.[/latex]
32. [latex]{\displaystyle\int }_{t=\pi }^{0}b\sqrt{1-{ \cos }^{2}t}×(\text{−}a \sin t)dt={\displaystyle\int }_{t=0}^{\pi }ab{ \sin }^{2}tdt[/latex]
33. [latex]f(t)=2 \cos (3t)- \cos (2t);{\displaystyle\int }_{0}^{\pi \text{/}2}(2 \cos (3t)- \cos (2t))=-\frac{2}{3}[/latex]

Integrals Involving Exponential and Logarithmic Functions

1. [latex]\frac{1}{9}{x}^{3}(\text{ln}({x}^{3})-1)+C[/latex]
2. [latex]2\sqrt{x}(\text{ln}x-2)+C[/latex]
3. [latex]{\displaystyle\int }_{0}^{\text{ln}x}{e}^{t}dt={e}^{t}{|}_{0}^{\text{ln}x}={e}^{\text{ln}x}-{e}^{0}=x-1[/latex]
4. [latex]-\frac{1}{3}\text{ln}( \sin (3x)+ \cos (3x))[/latex]
5. [latex]-\frac{1}{2}\text{ln}| \csc ({x}^{2})+ \cot ({x}^{2})|+C[/latex]
6. [latex]-\frac{1}{2}{(\text{ln}( \csc x))}^{2}+C[/latex]
7. [latex]\frac{1}{3}\text{ln}(\frac{26}{7})[/latex]
8. [latex]y-2\text{ln}|y+1|+C[/latex]
9. [latex]\text{ln}| \sin x- \cos x|+C[/latex]
10. [latex]-\frac{1}{3}{(1-(\text{ln}{x}^{2}))}^{3\text{/}2}+C[/latex]
11. Exact solution: [latex]\frac{e-1}{e},{R}_{50}=0.6258.[/latex] Since [latex]f[/latex] is decreasing, the right endpoint estimate underestimates the area.
12. Exact solution: [latex]\frac{2\text{ln}(3)-\text{ln}(6)}{2},{R}_{50}=0.2033.[/latex] Since [latex]f[/latex] is increasing, the right endpoint estimate overestimates the area.
13. Exact solution: [latex]-\frac{1}{\text{ln}(4)},{R}_{50}=-0.7164.[/latex] Since [latex]f[/latex] is increasing, the right endpoint estimate overestimates the area (the actual area is a larger negative number).

Integrals Resulting in Inverse Trigonometric

1. [latex]{ \sin }^{-1}x{|}_{0}^{\sqrt{3}\text{/}2}=\frac{\pi }{3}[/latex]
2. [latex]{ \tan }^{-1}x{|}_{\sqrt{3}}^{1}=-\frac{\pi }{12}[/latex]
3. [latex]{ \sec }^{-1}x{|}_{1}^{\sqrt{2}}=\frac{\pi }{4}[/latex]