Derivatives of Trigonometric Functions
For the following exercises (1-5), find [latex]\frac{dy}{dx}[/latex] for the given functions.
- [latex]y=x^2- \sec x+1[/latex]
- [latex]y=x^2 \cot x[/latex]
- [latex]y=\dfrac{\sec x}{x}[/latex]
- [latex]y=(x+ \cos x)(1- \sin x)[/latex]
- [latex]y=\dfrac{1- \cot x}{1+ \cot x}[/latex]
For the following exercises (6-8), find the equation of the tangent line to each of the given functions at the indicated values of [latex]x[/latex]. Then use a calculator to graph both the function and the tangent line to ensure the equation for the tangent line is correct.
- [latex]f(x)=−\sin x, \,\,\, x=0[/latex]
- [latex]f(x)=1+ \cos x, \,\,\, x=\frac{3\pi}{2}[/latex]
- [latex]f(x)=x^2- \tan x, \,\,\, x=0[/latex]
For the following exercises (9-11), find [latex]\frac{d^2 y}{dx^2}[/latex] for the given functions.
- [latex]y=x \sin x- \cos x[/latex]
- [latex]y=x-\frac{1}{2} \sin x[/latex]
- [latex]y=2 \csc x[/latex]
For the following exercises (12-15), solve each problem.
- Find all [latex]x[/latex] values on the graph of [latex]f(x)=-3 \sin x \cos x[/latex] where the tangent line is horizontal.
- Let [latex]f(x)= \cot x[/latex]. Determine the point(s) on the graph of [latex]f[/latex] for [latex]0 < x < 2\pi[/latex] where the tangent line is parallel to the line [latex]y=-2x[/latex].
- Let the position of a swinging pendulum in simple harmonic motion be given by [latex]s(t)=a \cos t+b \sin t[/latex] where [latex]a[/latex] and [latex]b[/latex] are constants, [latex]t[/latex] measures time in seconds, and [latex]s[/latex] measures position in centimeters, If the position is [latex]0[/latex]cm and the velocity is [latex]3[/latex]cm/s when [latex]t=0[/latex], find the values of [latex]a[/latex] and [latex]b[/latex].
- The number of hamburgers sold at a fast-food restaurant in Pasadena, California, is given by [latex]y=10+5 \sin x[/latex] where [latex]y[/latex] is the number of hamburgers sold and [latex]x[/latex] represents the number of hours after the restaurant opened at 11 a.m. until 11 p.m., when the store closes. Find [latex]y^{\prime}[/latex] and determine the intervals where the number of burgers being sold is increasing.
For the following exercises (16-18), find the requested higher-order derivative for the given functions.
- [latex]\frac{d^3 y}{dx^3}[/latex] of [latex]y=3 \cos x[/latex]
- [latex]\frac{d^4 y}{dx^4}[/latex] of [latex]y=5 \cos x[/latex]
- [latex]\frac{d^3 y}{dx^3}[/latex] of [latex]y=x^{10}- \sec x[/latex]
The Chain Rule
For the following exercises (1-3), given [latex]y=f(u)[/latex] and [latex]u=g(x)[/latex], find [latex]\frac{dy}{dx}[/latex] by using Leibniz’s notation for the chain rule: [latex]\frac{dy}{dx}=\frac{dy}{du}\frac{du}{dx}[/latex].
- [latex]y=6u^3, \,\,\, u=7x-4[/latex]
- [latex]y= \cos u, \,\,\, u=\dfrac{−x}{8}[/latex]
- [latex]y=\sqrt{4u+3}, \,\,\, u=x^2-6x[/latex]
For each of the following exercises (4-7),
- decompose each function in the form [latex]y=f(u)[/latex] and [latex]u=g(x)[/latex], and
- find [latex]\frac{dy}{dx}[/latex] as a function of [latex]x[/latex].
- [latex]y=(3x^2+1)^3[/latex]
- [latex]y=\left(\dfrac{x}{7}+\dfrac{7}{x}\right)^7[/latex]
- [latex]y= \csc (\pi x+1)[/latex]
- [latex]y=-6 \sin^{-3} x[/latex]
For the following exercises (8-12), find [latex]\frac{dy}{dx}[/latex] for each function.
- [latex]y=(5-2x)^{-2}[/latex]
- [latex]y=(2x^3-x^2+6x+1)^3[/latex]
- [latex]y=(\tan x+ \sin x)^{-3}[/latex]
- [latex]y= \sin (\cos 7x)[/latex]
- [latex]y= \cot^3 (4x+1)[/latex]
For the following exercises (13-16), use the information in the following table to find [latex]h^{\prime}(a)[/latex] at the given value for [latex]a[/latex].
| [latex]x[/latex] | [latex]f(x)[/latex] | [latex]f^{\prime}(x)[/latex] | [latex]g(x)[/latex] | [latex]g^{\prime}(x)[/latex] |
|---|---|---|---|---|
| [latex]0[/latex] | [latex]2[/latex] | [latex]5[/latex] | [latex]0[/latex] | [latex]2[/latex] |
| [latex]1[/latex] | [latex]1[/latex] | [latex]−2[/latex] | [latex]3[/latex] | [latex]0[/latex] |
| [latex]2[/latex] | [latex]4[/latex] | [latex]4[/latex] | [latex]1[/latex] | [latex]−1[/latex] |
| [latex]3[/latex] | [latex]3[/latex] | [latex]−3[/latex] | [latex]2[/latex] | [latex]3[/latex] |
- [latex]h(x)=f(g(x)); \,\,\, a=0[/latex]
- [latex]h(x)=(x^4+g(x))^{-2}; \,\,\, a=1[/latex]
- [latex]h(x)=f(x+f(x)); \,\,\, a=1[/latex]
- [latex]h(x)=g(2+f(x^2)); \, a=1[/latex]
For the following exercises (17-23), solve each problem.
- Let [latex]y=(f(x)+5x^2)^4[/latex] and suppose that [latex]f(-1)=-4[/latex] and [latex]\frac{dy}{dx}=3[/latex] when [latex]x=-1[/latex]. Find [latex]f^{\prime}(-1)[/latex]
- Find the equation of the tangent line to [latex]y=−\sin \left(\dfrac{x}{2}\right)[/latex] at the origin. Use a calculator to graph the function and the tangent line together.
- Find the [latex]x[/latex]-coordinates at which the tangent line to [latex]y=\left(x-\dfrac{6}{x}\right)^8[/latex] is horizontal.
- The position function of a freight train is given by [latex]s(t)=100(t+1)^{-2}[/latex], with [latex]s[/latex] in meters and [latex]t[/latex] in seconds. At time [latex]t=6[/latex] s, find the train’s
- velocity and
- acceleration.
- Using a. and b. is the train speeding up or slowing down?
- The total cost to produce [latex]x[/latex] boxes of Thin Mint Girl Scout cookies is [latex]C[/latex] dollars, where [latex]C=0.0001x^3-0.02x^2+3x+300[/latex]. In [latex]t[/latex] weeks production is estimated to be [latex]x=1600+100t[/latex] boxes.
- Find the marginal cost [latex]C^{\prime}(x)[/latex].
- Use Leibniz’s notation for the chain rule, [latex]\frac{dC}{dt}=\frac{dC}{dx} \cdot \frac{dx}{dt}[/latex], to find the rate with respect to time [latex]t[/latex] that the cost is changing.
- Use b. to determine how fast costs are increasing when [latex]t=2[/latex] weeks. Include units with the answer.
- The formula for the volume of a sphere is [latex]S=\frac{4}{3}\pi r^3[/latex], where [latex]r[/latex] (in feet) is the radius of the sphere. Suppose a spherical snowball is melting in the sun.
- Suppose [latex]r=\dfrac{1}{(t+1)^2}-\dfrac{1}{12}[/latex] where [latex]t[/latex] is time in minutes. Use the chain rule [latex]\frac{dS}{dt}=\frac{dS}{dr} \cdot \frac{dr}{dt}[/latex] to find the rate at which the snowball is melting.
- Use a. to find the rate at which the volume is changing at [latex]t=1[/latex] min.
- The depth (in feet) of water at a dock changes with the rise and fall of tides. The depth is modeled by the function [latex]D(t)=5 \sin \left(\dfrac{\pi}{6} t-\dfrac{7\pi}{6}\right)+8[/latex], where [latex]t[/latex] is the number of hours after midnight. Find the rate at which the depth is changing at 6 a.m.
Derivatives of Inverse Functions
For the following exercises (1-2), use the graph of [latex]y=f(x)[/latex] to
- sketch the graph of [latex]y=f^{-1}(x)[/latex], and
- use part (a) to estimate [latex](f^{-1})^{\prime}(1)[/latex].
For the following exercises (3-4), use the functions [latex]y=f(x)[/latex] to find
- [latex]\frac{df}{dx}[/latex] at [latex]x=a[/latex] and
- [latex]x=f^{-1}(y)[/latex].
- Then use part (b) to find [latex]\frac{df^{-1}}{dy}[/latex] at [latex]y=f(a)[/latex].
- [latex]f(x)=2x^3-3, \,\,\ x=1[/latex]
- [latex]f(x)= \sin x, \,\,\ x=0[/latex]
For each of the following functions (5-7), find [latex](f^{-1})^{\prime}(a)[/latex].
- [latex]f(x)=x^3+2x+3, \,\,\ a=0[/latex]
- [latex]f(x)=x-\dfrac{2}{x}, \,\,\ x<0, \,\,\ a=1[/latex]
- [latex]f(x)= \tan x+3x^2, \,\,\ a=0[/latex]
For each of the given functions (8-9) [latex]y=f(x)[/latex],
- find the slope of the tangent line to its inverse function [latex]f^{-1}[/latex] at the indicated point [latex]P[/latex], and
- find the equation of the tangent line to the graph of [latex]f^{-1}[/latex] at the indicated point.
- [latex]f(x)=\sqrt{x-4}, \,\,\ P(2,8)[/latex]
- [latex]f(x)=−x^3-x+2, \,\,\ P(-8,2)[/latex]
For the following exercises (10-14), find [latex]\frac{dy}{dx}[/latex] for the given function.
- [latex]y= \sin^{-1}(x^2)[/latex]
- [latex]y= \sec^{-1}\left(\dfrac{1}{x}\right)[/latex]
- [latex]y=(1 + \tan^{-1} x)^3[/latex]
- [latex]y=\dfrac{1}{\tan^{-1}(x)}[/latex]
- [latex]y= \cot^{-1} \sqrt{4-x^2}[/latex]
For the following exercises (15-17), use the given values to find [latex](f^{-1})^{\prime}(a)[/latex].
- [latex]f(\pi)=0, \,\,\ f^{\prime}(\pi)=-1, \,\,\ a=0[/latex]
- [latex]f(\frac{1}{3})=-8, \,\,\ f^{\prime}(\frac{1}{3})=2, \,\,\ a=-8[/latex]
- [latex]f(1)=-3, \,\,\ f^{\prime}(1)=10, \,\,\ a=-3[/latex]
For the following exercises (18-20), complete all parts of each question. You may utilize calculators or any technological aids to facilitate your calculations.
- The position of a moving hockey puck after [latex]t[/latex] seconds is [latex]s(t)= \tan^{-1} t[/latex] where [latex]s[/latex] is in meters.
- Find the velocity of the hockey puck at any time [latex]t[/latex].
- Find the acceleration of the puck at any time [latex]t[/latex].
- Evaluate a. and b. for [latex]t=2,4[/latex], and 6 seconds.
- What conclusion can be drawn from the results in c.?
- A pole stands 75 feet tall. An angle [latex]\theta[/latex] is formed when wires of various lengths of [latex]x[/latex] feet are attached from the ground to the top of the pole, as shown in the following figure. Find the rate of change of the angle [latex]\frac{d\theta}{dx}[/latex] when a wire of length 90 feet is attached.

- A local movie theater with a 30-foot-high screen that is 10 feet above a person’s eye level when seated has a viewing angle [latex]\theta[/latex] (in radians) given by [latex]\theta = \cot^{-1}\left(\frac{x}{40}\right)- \cot^{-1}\left(\frac{x}{10}\right)[/latex],
where [latex]x[/latex] is the distance in feet away from the movie screen that the person is sitting, as shown in the following figure.
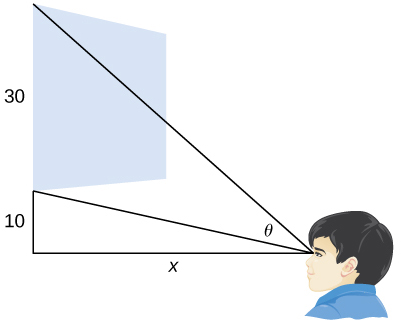
- Find [latex]\frac{d\theta}{dx}[/latex].
- Evaluate [latex]\frac{d\theta}{dx}[/latex] for [latex]x=5,10,15[/latex], and [latex]20[/latex].
- Interpret the results in b.
- Evaluate [latex]\frac{d\theta}{dx}[/latex] for [latex]x=25,30,35[/latex], and [latex]40[/latex]
- Interpret the results in d. At what distance [latex]x[/latex] should the person sit to maximize his or her viewing angle?
Implicit Differentiation
For the following exercises (1-5), use implicit differentiation to find [latex]\frac{dy}{dx}[/latex].
- [latex]6x^2+3y^2=12[/latex]
- [latex]3x^3+9xy^2=5x^3[/latex]
- [latex]y\sqrt{x+4}=xy+8[/latex]
- [latex]y \sin(xy)=y^2+2[/latex]
- [latex]x^3 y+xy^3=-8[/latex]
For the following exercises (6-8), find the equation of the tangent line to the graph of the given equation at the indicated point. Use a calculator or computer software to graph the function and the tangent line.
- [latex]x^2 y^2+5xy=14, \,\,\, (2,1)[/latex]
- [latex]xy^2 + \sin(\pi y)-2x^2=10, \,\,\, (2,-3)[/latex]
- [latex]xy+ \sin (x)=1, \,\,\, (\frac{\pi}{2},0)[/latex]
For the following exercises (9-13), solve each problem.
- For the equation [latex]x^2+2xy-3y^2=0[/latex],
- Find the equation of the normal to the tangent line at the point [latex](1,1)[/latex].
- At what other point does the normal line in a. intersect the graph of the equation?
- For the equation [latex]x^2+xy+y^2=7[/latex],
- Find the [latex]x[/latex]-intercept(s).
- Find the slope of the tangent line(s) at the [latex]x[/latex]-intercept(s).
- What does the value(s) in b. indicate about the tangent line(s)?
- Find the equation of the tangent line to the graph of the equation [latex]\tan^{-1}(x+y)=x^2+\frac{\pi}{4}[/latex] at the point [latex](0,1)[/latex].
- The number of cell phones produced when [latex]x[/latex] dollars is spent on labor and [latex]y[/latex] dollars is spent on capital invested by a manufacturer can be modeled by the equation [latex]60x^{\frac{3}{4}}y^{\frac{1}{4}}=3240[/latex].
- Find [latex]\frac{dy}{dx}[/latex] and evaluate at the point [latex](81,16)[/latex].
- Interpret the result of a.
- The volume of a right circular cone of radius [latex]x[/latex] and height [latex]y[/latex] is given by [latex]V=\frac{1}{3}\pi x^2 y[/latex]. Suppose that the volume of the cone is [latex]85\pi \, \text{cm}^3[/latex]. Find [latex]\frac{dy}{dx}[/latex] when [latex]x=4[/latex] and [latex]y=16[/latex].
For the following exercise, consider a closed rectangular box with a square base with side [latex]x[/latex] and height [latex]y[/latex].
- If the surface area of the rectangular box is [latex]78[/latex] square feet, find [latex]\frac{dy}{dx}[/latex] when [latex]x=3[/latex] feet and [latex]y=5[/latex] feet.
For the following exercise, use implicit differentiation to determine [latex]y^{\prime}[/latex]. Does the answer agree with the formulas we have previously determined?
- [latex]x= \cos y[/latex]
Derivatives of Exponential and Logarithmic Functions
For the following exercises (1-8), find [latex]f^{\prime}(x)[/latex] for each function.
- [latex]f(x)=x^2 e^x[/latex]
- [latex]f(x)=e^{x^3 \ln x}[/latex]
- [latex]f(x)=\dfrac{e^x-e^{−x}}{e^x+e^{−x}}[/latex]
- [latex]f(x)=2^{4x}+4x^2[/latex]
- [latex]f(x)=x^{\pi} \cdot \pi^x[/latex]
- [latex]f(x)=\ln \sqrt{5x-7}[/latex]
- [latex]f(x)=\log(\sec x)[/latex]
- [latex]f(x)=2^x \cdot \log_3 7^{x^2-4}[/latex]
For the following exercises (9-12), use logarithmic differentiation to find [latex]\frac{dy}{dx}[/latex].
- [latex]y=(\sin 2x)^{4x}[/latex]
- [latex]y=x^{\log_2 x}[/latex]
- [latex]y=x^{\cot x}[/latex]
- [latex]y=x^{-\frac{1}{2}}(x^2+3)^{\frac{2}{3}}(3x-4)^4[/latex]
For the following exercises (13-16), solve each problem.
- Find the equation of the line that is normal to the graph of [latex]f(x)=x \cdot 5^x[/latex] at the point where [latex]x=1[/latex]. Graph both the function and the normal line.
- Consider the function [latex]y=x^{\frac{1}{x}}[/latex] for [latex]x>0[/latex].
- Determine the points on the graph where the tangent line is horizontal.
- Determine the intervals where [latex]y^{\prime}>0[/latex] and those where [latex]y^{\prime}<0[/latex].
- The population of Toledo, Ohio, in 2000 was approximately [latex]500,000[/latex]. Assume the population is increasing at a rate of [latex]5\%[/latex] per year.
- Write the exponential function that relates the total population as a function of [latex]t[/latex].
- Use a. to determine the rate at which the population is increasing in [latex]t[/latex] years.
- Use b. to determine the rate at which the population is increasing in [latex]10[/latex] years.
- The number of cases of influenza in New York City from the beginning of 1960 to the beginning of 1961 is modeled by the function
[latex]N(t)=5.3e^{0.093t^2-0.87t}, \, (0\le t\le 4)[/latex],
where [latex]N(t)[/latex] gives the number of cases (in thousands) and [latex]t[/latex] is measured in years, with [latex]t=0[/latex] corresponding to the beginning of 1960.
- Show work that evaluates [latex]N(0)[/latex] and [latex]N(4)[/latex]. Briefly describe what these values indicate about the disease in New York City.
- Show work that evaluates [latex]N^{\prime}(0)[/latex] and [latex]N^{\prime}(3)[/latex]. Briefly describe what these values indicate about the disease in New York City.
For the following exercises (17-20), use the population of New York City from 1790 to 1860, given in the following table.
| Years since 1790 | Population |
| [latex]0[/latex] | [latex]33,131[/latex] |
| [latex]10[/latex] | [latex]60,515[/latex] |
| [latex]20[/latex] | [latex]96,373[/latex] |
| [latex]30[/latex] | [latex]123,706[/latex] |
| [latex]40[/latex] | [latex]202,300[/latex] |
| [latex]50[/latex] | [latex]312,710[/latex] |
| [latex]60[/latex] | [latex]515,547[/latex] |
| [latex]70[/latex] | [latex]813,669[/latex] |
- Using a computer program or a calculator, fit a growth curve to the data of the form [latex]p=ab^t[/latex].
- Using the exponential best fit for the data, write a table containing the derivatives evaluated at each year.
- Using the exponential best fit for the data, write a table containing the second derivatives evaluated at each year.
- Using the tables of first and second derivatives and the best fit, answer the following questions:
- Will the model be accurate in predicting the future population of New York City? Why or why not?
- Estimate the population in 2010. Was the prediction correct from a.?

